It was an honour to be elected. I was on the faculty at Inchfield, and seized the opportunity to work under specialist in topologic geometry, Professor Knowlton. Five years later, I was working on a level nearly lateral to his, which earned me the invitation to an informal gathering in his garden. This is where he and a select few would deliberate over nominees for the prestigious Roberts Prize in Mathematics, and who would be awarded its substantial cash prize. Seeing as that year, it was Knowlton’s privilege to judge.
Having been to Knowlton’s house before once or twice, I’d a cursory acquaintance with his unkempt hedges, substantial brick residence, and an older son who had since entered a foreign university. Knowlton seldom mentioned his younger son, who was mentally deficient.
We settled at a mosaic table on a piazza, near enough to the French doors that Mrs. Knowlton could handily supply us with coffee and its accompaniments. As I anticipated, Dr. Fuller kicked off the meeting by hammering his preference for a member of his own staff. And though the lad in question had been nominated by someone else, we were gratified when Morris, whose field is probability, grilled Fuller. “Yes, yes, of course he’s all those things, but isn’t he also in fact, your godson?”
The laughter that ensued provided Sorensen an opportunity to introduce his own protégé, an emeritus in Arizona whose research with Euler circuits had thus far attracted only local attention. Sorensen’s a sucker for obscure underdogs. For example, from an array of composers that including Sorensen, no more than six other human beings have ever heard of, like one would a boutonnière, he’ll select his current favorite. He amuses me so much, that I was quite preoccupied when from around the corner came Knowlton’s younger son, to sidle up behind me.
Slight, and fair haired, Donald was perhaps sixteen at the time. I suppose he chose me because I was the youngest at the table, and because I always greeted him with a smile. He touched my collar and whispered loudly, “Mis’er Irving, come with me. I want to show you something.”
“Later, Donald” I murmured. But his expression, eager to the point of pain, got me off my chair, and excusing myself. However the frowning Knowlton was quick to chastise his son. “Donny, go away. Mr. Irving and I are talking.”
Donald displayed a particular kind of fear, hurt, and anger which alarmed me. His expression reminded me of a childhood playmate whose father drank. But for the moment reassured by the bland face of Prof. Knowlton, I followed Donald.
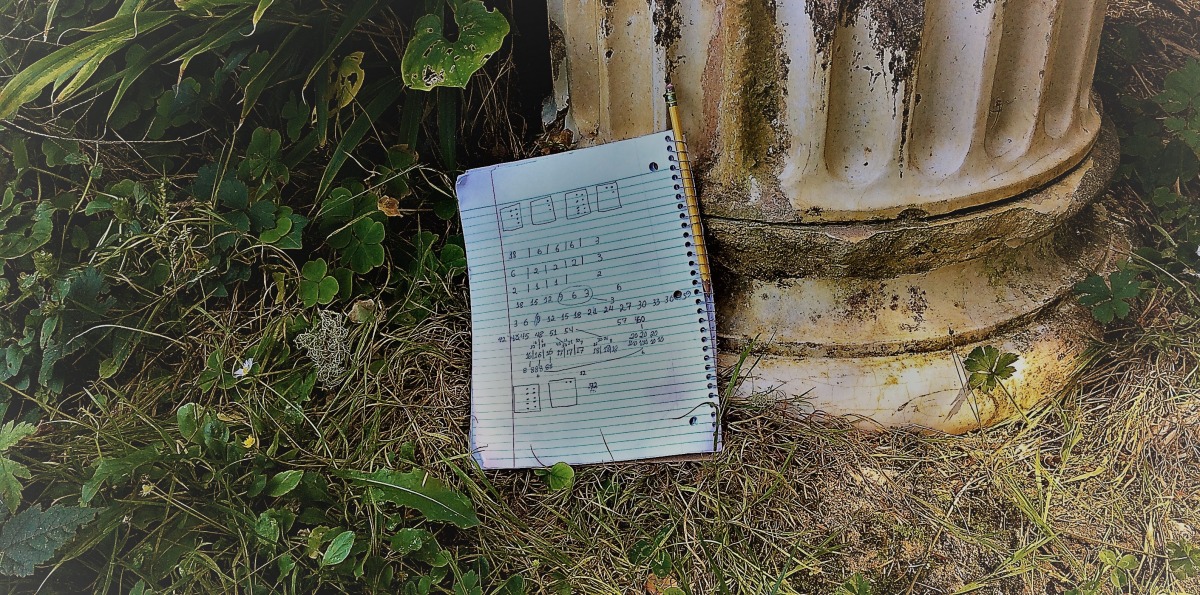
The boy led me back around the corner he’d come from, and via a side-door, in to the house. He then took me up a flight of stairs to his room, which, bare of the expected zoological, mechanical, or academic clutter, was very tidy. And taking from under his bed, a battered spiral notebook, he passed it to me.
I leafed through the early pages full of penciled numbers, by no means neat, but not illegible. Some basic problems in addition and subtraction. But impatient, he snatched the book and thumbing deeply into it, then handed it back, pointing to an area on the left-hand page. Obedient, I looked only to find before me, his wobbly notation of the Fibonacci Sequence. That plaything of the best minds in each century, encrypted by Nature in cauliflowers and pine cones—1, 2, 3, 5, 8, 13, 21…
Smiling I pointed to the sequence, and when Imurmured its terms, his own face spread with corresponding joy. “Yes, one an’ two, then two an’ three, then three an’ five…”
Noting that his written sequence ended at 89, I pointed to the last terms. “Fifty-five and eighty-nine?” Blinking, he grimaced, and pressing fingers, which twitched, to his jaw, he retreated. I waited while, with his skull in both hands, he sat on the smooth white bed.
“A hun’red and forty-four!”
I tried to smile, suddenly aching that this devoted mathematician should have to strain so hard to take the first steps of the science. He was still frowning and holding his head, as I continued to leaf through the later pages.
“A hun’red and forty-four, Mis’er Irving, is twelve twelves,” said Donald. Dismounting from the bed, he took the book, for the purpose of indicating another sequence: 1, 4, 9, 16, 25…
Again, I was arrested by the point where the sequence stopped.
“Fourteen times fourteen?” I murmured, almost at once regretting that I had. Retrieving the book, with a fresh frown, he retreated to the bed, and began drawing little squares and dotting them. I bent over him to see and realized that he was solving the problem through a crude yet ingenious system of incremental multiplication, similar to a written abacus, which I could only imagine he had invented himself. He seemed to have no notion of the usefulness of place value and columnar operations.
“A hun’red and ninety-six,” he produced at last, straightening.
“Who taught you to do this?”
He blinked.
“Donald, did your mother teach you to add fourteen fourteens like that?”
“No.”
“The one, two, three, five, eight, did your mother teach you that? Did anyone teach you that?”
“No.”
“Would you like to have someone teach you about numbers?”
“No,” said Donald.
“Have they tried?”
“My math is different,” said Donald.
I’’m not proud of it, but to be sure that Fuller wasn’t getting anywhere with his prodigy I needed to go back downstairs, and that’s where I went.
Though Knowlton’s sarcastic appraisal lowered my estimation later, Gening, a statistician for whom I had the greatest respect in those days, was holding the floor when I arrived. In fact, as he laid out the merits of a statistician in Washington, it occurred to me that he possessed all those qualifications himself, only more so. Statistic analysis has never been my strength, and I have, perhaps exaggerated, respect for people who master standard deviation at an earlier age than I did.
When Donald came up behind me again, his hand touched my collar just at the same moment that Knowlton snapped, “Donald!” Out-of-place against a noble old hawthorn hedge, the boy was wilting before my eyes, which prompted me to rise and, once more, follow him.
Donald did not take me upstairs, but only out of earshot. “Mis’er Irving,” he said, “You di’n’t stay for what I wanted to tell you. Daddy’s judging the Roberts Prize for math. I want to enter wit’out him knowing. I can’t enter if he knows, you know. It wouldn’t be fair to the others.”
“Donald, you have to be nominated, you see. That’s what those other mathematicians are here for today, to help your daddy pick somebody good out of the ones that have already been nominated.”
“But they haven’t picked yet, have they?”
“Well, no.”
“Then couldn’t you nominate me, Mis’er Irving?”
At this moment Ivy, the Knowltons’ maid, came in. “Mr. Irving, Mr. Knowlton begs you not to pay any mind to Donald. He’s been moody all week. Mr. Knowlton’s specially anxious to have your thoughts on the selection.”
I hadn’t known how much I’d been hoping to hear this until it presented itself.
“Certainly, Ivy. I’m coming.”
I had my own definite idea about a candidate, but wouldn’t have brought it up without this encouragement. Silently thankful for Donald’s interruption, I took my iron-filigree chair and began.
“What seems to me,” and gazing at each face, I saw how my sententious tone caught them by surprise, but they remained attentive to me, “is that we’ve an almost equal array of accomplishments before us. Who can say which achievement will really mean more to the science, and to progress. Which will really find its most useful expression, in the future? Burkhardt’s circuits? Pauley’s conchoidal surfaces? Who can tell? What we can estimate now, right here, is the human contribution, the dedication, the labor, that a particular candidate puts into their field. Begin with the expenditure of time. I happen to know that Tillson, for example…”
Every face turned to the French doors, behind which were sounds of struggle, Ivy’s breathless protests, and Donald’s urgent, partly muffled exclamations.
“Ivy! What’s the boy doing?” demanded Knowlton with an expression of stern distaste.
“Oh, he won’t… won’t… stay inside like you asked,” she answered.
“Donald!” barked Knowlton. “Stay inside, for heaven’s sake. Give me half an hour!… Ivy, tell him I’ll walk with him after…” and raising his watch,“three, we’ll go to the duck pond at three o’clock.”
“No!” Donald’s protest was clearly audible. “I have one thing to say to Mis’er Irving, one thing! Mis’er Irving don’t mind, ask him, he don’t!”
“Donald,” Knowlton’s voice adopted a tone I would not have defied as a boy, “Mr. Irving does mind. He is here on business. No, Irvie, please,” as I must have started to get up and go to the boy. “Donald, this isn’t like you. Why don’t you go upstairs and draw in your sketchbook for awhile?”
The inner rumpus subsided. Ivy must have persuaded Donald to go upstairs. Frightened that my little opportunity would be lost, I frantically tried to pick up my thread, but picked up something quite unconnected, as in my nervousness, I blurted it out.
“Knowlton, who teaches Donald his mathematics?”
The broad, avuncular face was caught with surprise. “Donald? No one! They tried, years ago. Kate tried so hard back then. Hired a specially trained teacher from the elementary school. A tutor from the staff at her own girls’ college, stewed over the times tables with him herself for hours. It was no use. I doubt he can do more than addition on his fingers. We gave up when he turned twelve.”
I stared into the mosaic tabletop, as I felt my face became bright red. It must have been the sun on my neck that let me feel it. Looking up, I saw what none of the others could. Donald leaning out of a second-floor window, and waving his notebook. He pointed at me, then at himself. and nodded.
“Mr. Irving,” Benedict’s smooth, cultured diction interrupted, “You were speaking of a ‘human contribution.’ Permit me to remind you that the true measure of the human contribution of a mathematician is his contribution to humans. The significance of discovery, be it scientific, mathematical, or any sort, lies exactly in the degree to which it can be appreciated and put to use by the human community. That is the purpose of the Roberts Prize. It is a social recognition, paid in hard social and economic currency, awarded in a structured scientific community.”
I was distracted by Donald disappearing into the window and slamming it shut.
“So that,” I rejoined weakly, “if one had to deliberate awarding either Newton or Leibnitz a prize for the discovery of calculus? The criterion wouldn’t be who had worked longer, or harder, or more independently. But only who published, got it out there, for human consumption, first.”
Benedict seemed taken aback, but soon replied,“Isn’t that, Irving, the only honest way?”
“But suppose we found a lost medieval manuscript that described calculus. One that had been lost since it was made, that had never done a soul any good. Would it be a scientific achievement?”
Knowlton, of all of them, seemed readiest to agree. “Benedict! Think, man! A medieval Newton!”
I looked up and saw a light, that pensive face regarding me through the window. The head that independently endeavored in a science which I suppose had been a source of torment to him. The head which produced that little system of symbolic multiplication, by a labor I simply couldn’t imagine.
“No,” conceded Knowlton, laying his hands on the mosaic tabletop. “You’re right, Benny. It’s a social enterprise. Art is in the eye of the beholder.” He turned to Fuller. “Tell me again about the algorithm Beckridge used.”
“Bother the Roberts Prize,” I grumbled. And it is then, that I left my chair.